For correct assessment and adjustment of the vibration equipment, first the calculation of the centrifugal force is necessary. It activates the motion of the every separate particle of the mass which has to be moved.
Is the centrifugal force too small, the particle will not move and will remain at it’s objectionable state. Is the centrifugal force too high, e.g. for compacting undesirable motions are caused besides the necessary compaction.
To determine the centrifugal force, the mass mu of the eccentric disc, the distance e from the centre of gravity the centre of motion, and the frequency of mechanical vibration fm are important factors.
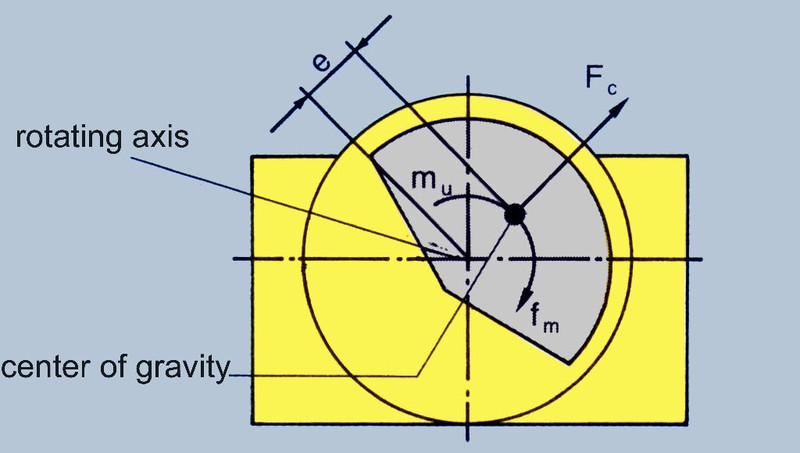
- Fig. 8: Formation of working moment Mu = mu ⋅ e
Fc = mu ⋅ e ⋅ ω2 / 1000
ω = 2 π ⋅ fm
Fc - centrifugal force in kN
mu - mass of the eccentric disc in kg
e - distance from the centre of gravity to the centre of motion in m
fm - mechanical frequency in 1/s
ω - angular speed of the eccentric disc in 1/s
In order to make the calculation easier just the minimum and maximum values of the centrifugal force at nominal (synchronous) speed are being taken.The synchronous speed of a three-phase asynchronous motor is calculated from the electrical mains frequency and the number of pole pairs.
Motor Rotation Speed
ns = 60 ⋅ fel / p
ns - synchronous speed in 1/min
p - number of pole pairs
fel - electrical frequency
Under load the speed of a asynchronous motor is reduced by its slip. The result is the operating speed.
N =ns ⋅ (1-σ)
n - operating speed (mechanical frequency) in 1/min
σ - slip
Because of the slightly smaller operating speed the Fc must be reduced by the factor (1-σ)2.
The fact, that the three-phase asynchronous motor at load in comparison to idle speed decreases only slightly (by slip), is a decisive advantage. In practice the mass of the eccentric weights is unknown, known are mass m, which has to be set to vibration, and the acceleration a. For the different areas of application there are multiple experience values available. Therefore Fc (kN) is determined according the equation.
Calculation of the Centrifugal Force in Practice
Fc = m ⋅ a / 1000
m - the sum of the masses, which has to be set into vibration, in kg:
- the mass of the vibrator mR
- the mass of the vibration equipment mT
- 10-15% of the mass which has to be compacted ms
m = mR + mT + ms
a - acceleration in m/s2
In addition a characteristic value for rigidity and the resonance behaviour of the vibration equipment have to be considered.
The centrifugal force Fcsets the entire equipment, including the mass to be vibrated, to vibration. The vibration width s (double amplitude) at the equipment must not be too high; otherwise the vibration equipment can be damaged. Should measuring of the vibration amplitude not be possible, it has to be calculated. The acceleration a is either already known or has to be taken from table 4.
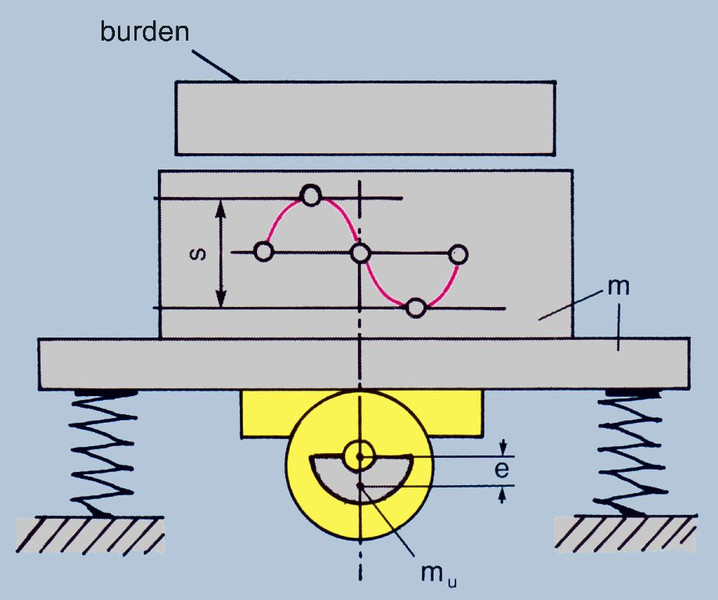
- Fig. 9: Vibration width [double amplitude] at a vibrating equipment during one motor revolution
Calculation of the Amplitude
s = a / 5,483 ⋅ nT2
s – Vibration width (double amplitude) in mm
a – scceleration in m/s2
nT = n/1000; n:vibration speed in 1/min
Influence of the Revolutions per Minute on the Centrifugal Force
Since frequency converters often are used to change the speed of the vibrator, it is important to know how the change of speed influences the double amplitude s and the centrifugal force.
Formula 1 shows that under the same conditions the centrifugal force changes quadratically to the speed. That means that for instance halving the revolution would result in quartering the centrifugal force. Contrary the centrifugal force is quadrupled when the speed is doubled. However, the height of the double amplitude s does not change.
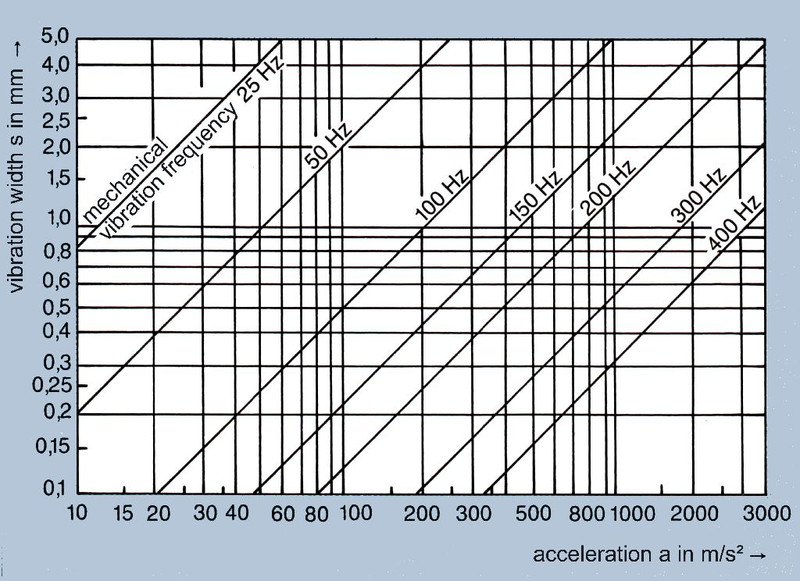
- Fig. 10: Relationship of centrifugal force, the amplitude and vibration speed
The double amplitude s results out of the relations
s/2 ⋅ m = mu e
MU = mue
s = (2 ⋅ Mu) / m ⋅ 10
Mu – eccentric torque; 2 ⋅ Mu = working moment MA in kgcm
m – sum of all moving masses in kg


 DE
DE ES
ES
